No matter what you install in your RC car or truck, when it comes to speed and run time it’s all about gearing. Understanding your vehicle’s gear ratio is a powerful tool when making changes at the track or around the backyard. Whether you’re aiming for longer run times or to set a land speed record against your friends, knowing what to change and busting out some simple math makes it easy to see how gearing works.

WHAT IS A “GEAR RATIO”?

Any two gears will have a “ratio”; if your car has a 20-tooth (20T) pinion and a 100-tooth (100T) spur gear, that’s a 20:100 ratio. We need to go one step further, however, and divide the number of teeth on the spur gear (100) by the number of teeth on the pinion (20). It’s easy:
100 ÷ 20 = 5

The gear ratio is 5:1 (five to one), and that means that the pinion must make five revolutions to turn the spur gear one revolution. It’s that simple.

You don’t need to spend a lot of money to add gearing options to your pit box. Robinson Racing offers convenient pinion “6 packs” for about $15. You can get even-number tooth counts (16-26T, #1050) or odd-number gears (15-25T, #1055).

PUTTING RATIOS TO WORK

Let’s say your friend’s car is running well; it has good acceleration and top speed, and the battery and motor aren’t being overworked. You have the same car, same motor and a battery of equal capacity, so you want to use his gear ratio. Let’s use the previous gearing example and say he has a 20T pinion and a 100T spur for a 5:1 ratio; however, you don’t have a 100T spur of your own; your only spur gear is a 125T. Which pinion should you use? Divide the spur-gear teeth by 5 (since you’re aiming for a 5:1 ratio) to find out:

125 ÷ 5 = 25

You should use a 25T pinion. Even though you have bigger gears than your friend, the ratio is still the same—5:1.

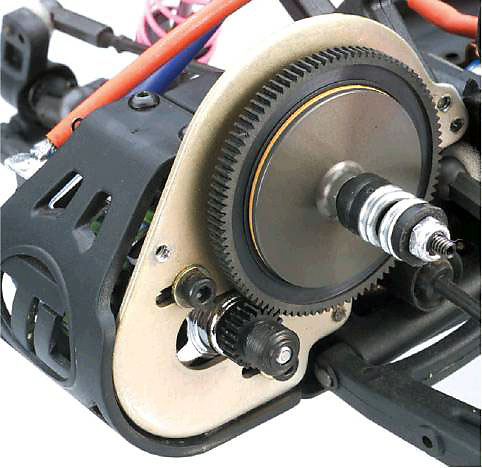
When most people talk about their car’s gear ratio, they are referring to the number of teeth on spur (large) gear and the pinion (small) gear.

CALCULATING TRANSMISSION RATIO

Any car with a gearbox has some type of internal gear reduction; most cars and trucks use a “3-gear” transmission that features a top or “input” gear, an idler gear and a bottom gear that spins the drive axles and incorporates the differential. To determine the transmission ratio, just divide the number of teeth on the diff (bottom) gear by the number of teeth on the top gear. For example, the gearbox shown below has a 20T top gear and 48T diff gear:

48 ÷ 20 = 2.4

The transmission ratio is 2.4:1. Once you have determined the transmission ratio, you can match your gear ratio to anyone else’s. Here’s how it works:

Let’s say you’re using that 20T-pinion/100T-spur combo in the example gearbox that has a transmission ratio of 2.4:1. To find the total drive reduction of your transmission, spur gear and pinion combination, you must multiply the transmission ratio (2.4:1) by the pinion/spur ratio (5:1):

2.4 x 5 = 12

The total drive reduction is 12:1. That means the pinion must turn 12 times to turn the diff gear (and the rear wheels) one complete revolution. Armed with this knowledge, let’s match this setup to a car with a different ratio. Let’s say, it’s a 2.3:1 transmission ratio. Start by dividing the desired result (12) by 2.3:

12 ÷ 2.3 = 5.2

Now we know we need a pinion-/spur-gear ratio of 5.2:1. We can calculate that by dividing our spur-gear tooth count by 5.2. Let’s say we have a 100T spur:

100 ÷ 5.2 = 19.23

Great! If we had a 19.23T pinion, we’d be all set. Of course, gears don’t have fractional teeth (unless you break a tooth!). You can quit now and run a slightly lower gear ratio by installing a 19T pinion, or gear slightly taller with a 20T pinion. If you want that elusive 100% equal ratio, you’ll have to keep looking for a pinion/spur combo that delivers a 5.2:1 ratio. Instead of working back from the spur gear, try working from the pinion. A nice, even number should work; let’s try a 20T pinion. Just multiply it by the desired ratio:

5.2 x 20 = 104

And there it is: a 20T pinion and a 104T spur deliver a perfect 5.2 ratio. Multiply 5.2 by the tranny ratio (2.3), and you get

11.96

that’s about as close to the desired 12:1 ratio you can get.

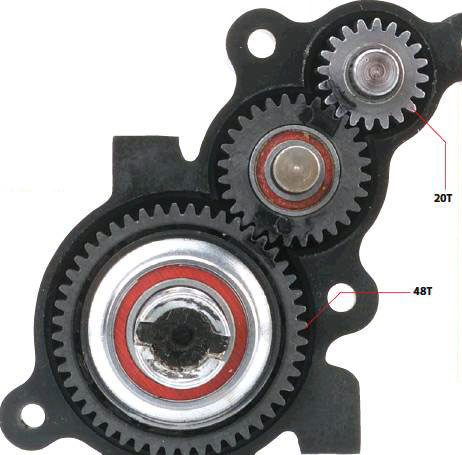
This transmission’s top gear has 20 teeth, and the diferential (bottom) gear has 48 teeth for a transmission ratio of 2.4:1. The idler (middle) gear has no effect on the total drive reduction; you can factor it into the equation, but it will cancel itself out.

DIRECT DRIVE Direct drive cars are ones that have no actual transmission to speak of. The pinion gear on the motor drives a spur gear, which seconds as the vehicles differential and is mounted directly (hence the name direct drive) to the axle. The final drive ratio of this type of vehicle is 1:1 (one revolution of “transmission” equals one revolution of the wheels) so as an effect of this, these types of cars are very fast. When finding the roll out of a direct-drive car you can skip the step of factoring in the transmission since its ratio is always 1. While they typically have higher top speeds, direct drive cars need to be very lightweight because more load is placed on the motor to move the vehicle the same distance. In this pan car, the motor drives the axle directly, hence the term “direct drive.” Right: In a shaft-driven car, the transmission ratio is determined by dividing the number of teeth on the differential’s ring gear by the number of teeth on the pinion attached to the driveshaft. BELT- AND SHAFT-DRIVE VEHICLES To determine the transmission ratio of a shaft- or belt-driven car, all you need to know is the tooth count of the pulleys (for a belt car)or the bevel gears (in a shaft car). For belt cars, simply count the number of teeth on the front or rear differential pulley, and divide by the number of teeth on the layshaft pulley (the layshaft is the shaft the spur gear is attached to). For shaft cars, the transmission ratio is calculated by dividing the number of teeth on the differential’s ring gear by the number of teeth on the bevel pinion that it mates with.

---

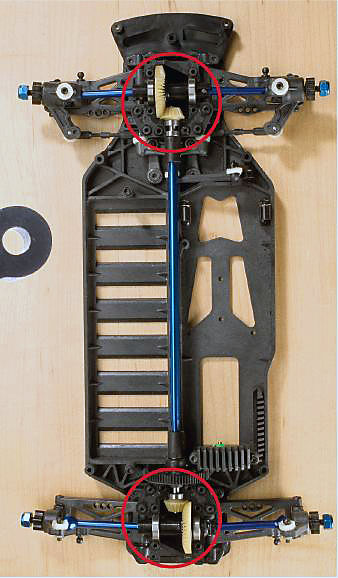
UNDERSTANDING GEAR PITCH A gear’s “pitch” refers to the size of its teeth; more specifically, the distance from a point on one tooth to the corresponding point on the next tooth. The higher the pitch number, the smaller the teeth. That seems backwards, but it makes sense when you consider how pitch is determined. Pitch is measured as the number of teeth that can fit around a disc with a diameter of 1 inch; for example, a tooth size that permits 48 teeth to encircle a 1-inch disc is said to be 48-pitch. To squeeze 64 teeth onto a 1-inch disc, the teeth must be made smaller to fit; that’s why 64-pitch gear teeth are finer than 48-pitch. The pitch of the gears in your car has no effect on their ratio; a 100T-spur/20T-pinion combination is a 5:1 ratio whether you use 32-, 48-, or 64-pitch gears. However, certain gear pitches are better suited to certain applications. For example: 32 PITCH This chunky gear pitch isn’t found on 1/10 electric vehicles much anymore, but gas cars, monster trucks and 4x4 short course trucks often use 32-pitch gears to cope with the heavy horsepower exerted on their transmissions. The only larger tooth profile in RC is “1 module,” a metric size that works out to 25.4 pitch. More on metric gears later. 48 PITCH Robinson Racing deserves credit for popularizing 48-pitch gears in off-road; until Rob Robinson developed the “in-between” pitch, racers had to choose between 32-pitch gears (durable, but limited in gearing options) or 64-pitch (plenty of gearing options, but the tiny teeth were easily stripped). The off-road standard, 48-pitch gears offer a good mix of ratio options and durability. 64 PITCH This is the pitch of choice among on-road racers mainly because of the huge number of available ratios and extra-smooth mesh of the very fine teeth. Since on-road cars don’t have to endure jumps and jolts, 64-pitch gears hold up well.

UNDERSTANDING METRIC PITCH You may have heard of “metric 64 pitch” or “metric 48 pitch.” Both types of gear are common to Japanese kits. The correct term for the pitch of these gears is “module”; 0.6-module gears are “metric 48” and are actually 42.3 pitch; “metric 64” gears are 0.4 module and are actually 63.5 pitch. Always use metric pinions with metric spurs; although the pitch is close to U.S. 48- and 64-pitch standards, they are not interchangeable.

---

ROLLOUT EXPLAINED Although they have tires instead of teeth, the drive wheels of your car or truck can be thought of as gears in the transmission. Think about it; if you take two identical cars with identical gear ratios and put small-diameter tires on one and large-diameter tires on another, the vehicle with larger tires will travel farther with each rotation of the wheels and is effectively geared taller than the small-tire car. To truly understand your car’s total gearing package, you must take the size of the drive wheels (or more accurately, their circumference) into account. The distance traveled by the tire in one rotation is its circumference. To measure it, mark the sidewall of the tire, roll it until the mark makes a complete rotation, then measure the distance. Or, calculate the circumference by multiplying pi (3.14) by the tire’s diameter. For example, if the diameter of your car’s drive tires is 5 inches, the circumference is: 3.14 x 5 in. = 15.7 in. If we divide that number by the total drive reduction of our example transmission (12:1), we get this result: 15.7 in. ÷ 12 = 1.31 in. That means the car travels forward 1.31 in. for each revolution of the pinion gear. In racer-speak, that’s the “rollout” for the car. No matter what we use for a car, or gears, or a transmission, any car set up with 1.31:1 rollout will be geared exactly the same as the example car.

In off-road racing, where tire diameters are relatively uniform, installing the same pinion and spur as another racer will net the same gear ratio. In oval and on-road carpet racing, tire diameter is determined by how much foam is trimmed off the tires, so rollout is used to compare gear ratios.

---

YOU CAN EASILY MATCH THE FINAL RATIO OF ONE VEHICLE TO ANOTHER TO GET THE SAME TOP SPEED, CORNERING POWER OR RUN TIME.

---

WHERE THE RUBBER HITS THE ROAD

By utilizing these mathematical formulas, you can easily calculate your vehicle’s gear, transmission, and overall drive ratio. With so many different styles of transmissions and various pitch gears, you can easily match the final ratio of one vehicle to another to get the same top speed, cornering power or run time. With some simple multiplication and division, the world of gear ratios can be unlocked for you.